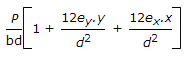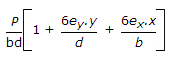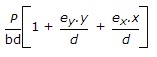Civil Engineering :: Theory of Structures
-
The forces in the members of simple trusses, may be analysed by
-
A bar l metre long and having its area of cross-section A, is subjected to a gradually applied tensile load W. The strain energy stored in the bar is
-
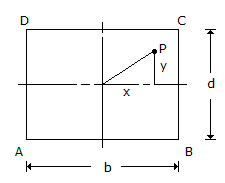
A rectangular column shown in the given figure carries a load P having eccentricities ex and ev along X and Y axes. The stress at any point (x, y) is
-
A simply supported beam which carries a uniformly distributed load has two equal overhangs. To have maximum B.M. produced in the beam least possible, the ratio of the length of the overhang to the total length of the beam, is
-
p = \( \frac { n^2EI } { 4L^2 } \)is the equation of Euler's crippling load, if
-
At yield point of a test piece, the material
-
For the close coil helical spring of the maximum deflection is
-
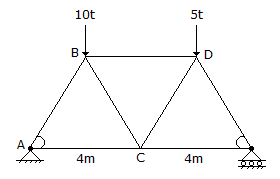
In the truss, the force in the member AC is
-
The moment of inertia of a triangular section (height h, base b) about its base, is
-
A steel bar 5 m x 50 mm is loaded with 250, 000 N. If the modulus of elasticity of the material is 0.2 MN/mm2 and Poisson's ratio is 0.25, the change in the volume of the bar is :


 Whatsapp
Whatsapp
 Facebook
Facebook