Discussion :: Surds and Indices
-
If x = 3 +2 √2, then the value of 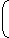
√X - 1 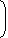
is: √X
Answer : Option B
Explanation :
[√x−1√X)2=X+1X−2
=(3+2√2)+ \frac { 1 } {(3+2€‹€‹€‹€‹€‹€‹ \sqrt{2)}} -2
=(3+2\sqrt{2})+ \frac { 1 } {(3+2€‹€‹€‹€‹€‹€‹ \sqrt{2)}} *\frac {( 3-€‹€‹€‹€‹2 \sqrt{2})} { (3-2\sqrt{2} )} -2
=(3+2\sqrt{2})+(3-2\sqrt{2})-2
=4
[\sqrt{X}-\frac { 1 } { \sqrt{X} }]=2
Be The First To Comment


 Whatsapp
Whatsapp
 Facebook
Facebook

